Symmedians and circumcircles

Classical Euclidean geometry is one of the oldest branches of mathematics, and in my opinion still as beautiful as ever. Here is a cute little geometric gemstone that was introduced to me recently by a fellow staff member at MOSP.

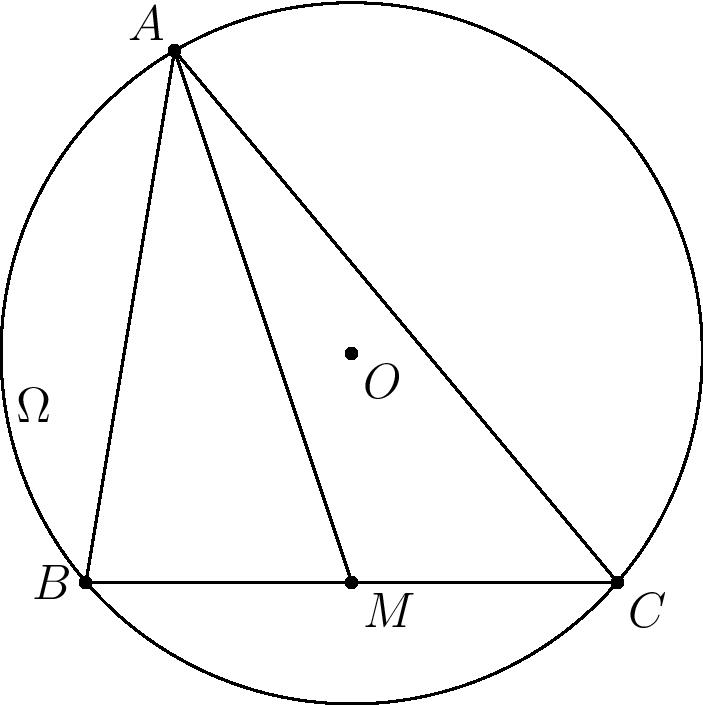
Consider a triangle $ABC$ drawn in the plane. Let $M$ be the midpoint of $BC$, and let $O$ be the circumcenter of triangle $ABC$, that is, the center of the circle $\Omega$ passing through $A$, $B$, and $C$.

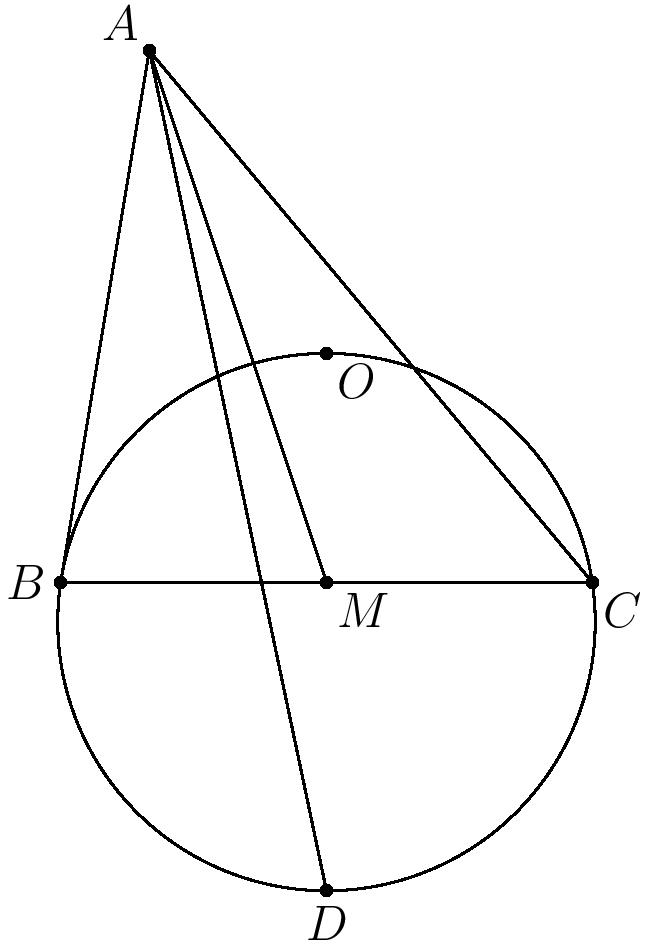
Now let $\omega$ the circumcircle of triangle $BOC$, and let $D$ be the point on $\omega$ outside of $ABC$ for which $MD$ is perpendicular to $BC$. Then it turns out that the line $AD$ can be obtained by reflecting the median $AM$ across the angle bisector of angle $A$! This reflection of $AM$ is called a symmedian of the triangle.

How can we go about proving this? Well, it suffices to show that angle $BAD$ is congruent to angle $CAM$. One way to go about showing angle measures are equal is by finding similar triangles. Triangles $AMC$ and $ABD$ look promising; let’s start by calculating angle $ABD$. This is equal to angle $B$ plus angle $CBD$, which is an inscribed angle on circle $\omega$. By the inscribed angle theorem (there is a beautiful interactive demonstration at that link!) angle $CBD$ is equal to angle $DOC$, which is half the arc $BC$ on circle $\Omega$. Thus angle $DOC$, and hence angle $CBD$, is equal to angle $A$ of the triangle.

We now have that angle $ABD$ is equal to angle $B$ plus angle $A$, which is $180-C$ (in degrees). So angle $ADB$ is $C-\theta$ where $\theta$ is angle $BAD$. Unfortunately this is not congruent to angle $C$, so triangles $AMC$ and $ABD$ are not similar.

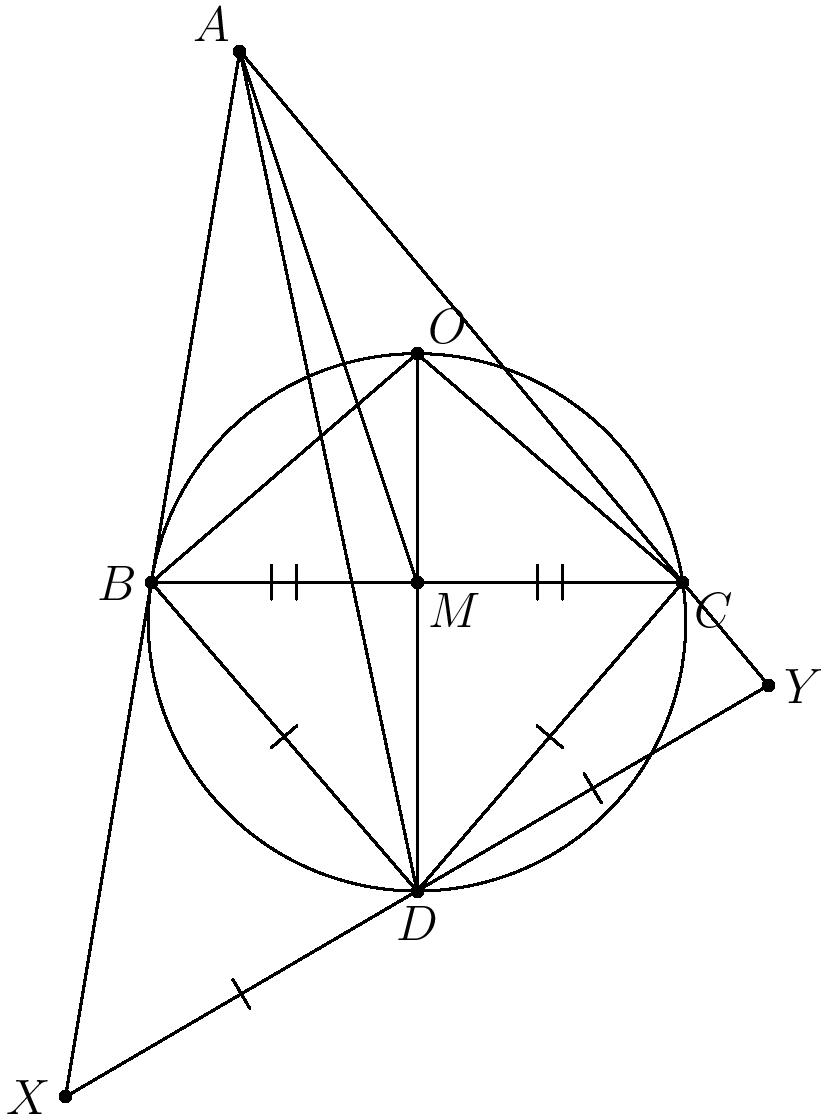
However, they’re rather close to being similar, in the following sense. Suppose we extend line $AB$ to a point $X$ for which $XD=DB$. Then triangle $BXD$ is isosceles, so angle $AXD$ is equal to angle $XBD$, which is supplementary to angle $ABD$. By our calculation above, angle $ABD$ is $A+B$ and so $AXD=XBD=C$. So, it is possible that triangles $ADX$ and $AMC$ are similar; this would suffice. (Notice also that this is a classic case of the falsity of “Angle Side Side” similarity - if it only takes an angle and two side lengths, then $ABD$ and $AMC$ would be similar too!)

Finally, we also extend $XD$ to meet the extension of $AC$ at $Y$. Then by looking at the sum of the angles in triangle $AXY$, we have that the angle at $Y$ is angle $B$. We also know by symmetry that angle $DCY$ is $B$, and so $DCY$ is isosceles and $DC=DY$. But $DC=DB$ as well since $D$ lies on the perpendicular bisector of $BC$. So \[DY=DC=DB=DX,\] and so $D$ is the midpoint of $XY$!

We are now almost done. Triangles $ABC$ and $AYX$ are now similar since all their angles are congruent, so we have \[\frac{AC}{AX}=\frac{BC}{XY}=\frac{2MC}{2DX}=\frac{MC}{DX},\] so by Side Angle Side similarity, triangles $AMC$ and $ADX$ are similar. So indeed, angle $BAD$ is equal to angle $MAC$.